Chance News 8
Oct 15 to Oct 30
table
| Treatment | Deaths per 1000 women |
|---|---|
| No mammograpy screening | 4 |
| Mammogrphy screening | 3 |
Quotation
One more fagot of these adamantine bandages, is, the new science of Statistics. It is a rule, that the most casual and extraordinary events -- if the basis of population is broad enough -- become matter of fixed calculation. It would not be safe to say when a captain like Bonaparte, a singer like Jenny Lind, or a navigator like Bowditch, would be born in Boston: but, on a population of twenty or two hundred millions, something like accuracy may be had. Ralph Waldo Emerson Fate
Forsooth
Here's another Forsooth from the October issue of RSS News.
Your'e more likely to die in a fire in Strathclyde than anywhere else in the country
11 May 2005
The Poisson distribution and the Supreme Court
The Poisson distribution and the Supreme Court
Journal of the American Statistical Association 31, no. 195 ,(1936), 376-80
W. Allen Wallis
Supreme Court Appointments as a Poisson distribution
American Journal of Political Science, 26, No.1, February 1982
S. Sidney Ulmer
This is not current news, but since Supreme Court appointments are in the news we felt that these articles might make an interesting class discussion.
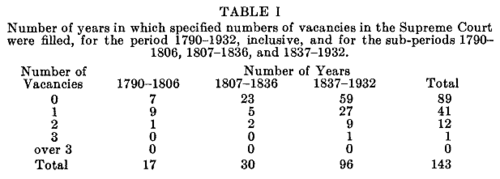
In 1936 statistician Allen Wallis suggested that a Poisson distribution can approximate the number of U.S. Supreme Court appointments in a given year. In his Table 1 Wallis provided the number appointed in each year over the intervals with different numbers of Justices on the Supreme Court: 1790-1806 (6) 1807-1836 (7), and 1837-1932 (9). Actually there were 10 in the years 1963 to 1967 which Wallis called "negligible exceptions."
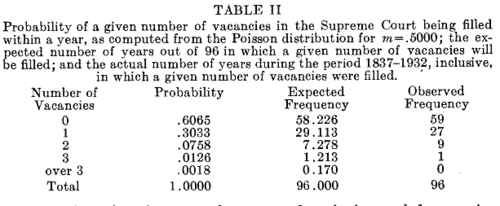
Wallis then restricts himself to the years 1837-1932 and finds that a Poisson distribution with mean .5 can approximate the number of court appointments in a year. His results are shown in his Figure II:
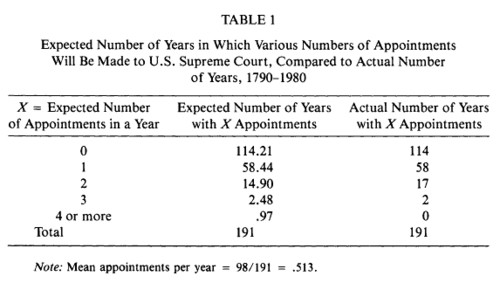
In his article, Sidney Ulmer updated the data to 1980 and also includes data from the early Supreme Courts with 6 and 7 members. Thus his data covers the years 1790--1980. His Table 1 compares the actual number of appointments in a year with the expected number of appointments assuming a Poisson distribution with mean 5.13.
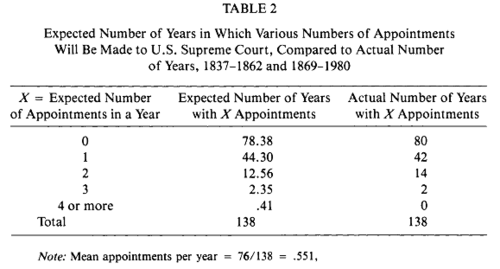
In his Table II Ulmer limits the data to the 9 member Supreme Courts: 1837 to 1862 and 1869-1980).
Testing the goodness of fits, Ulmer argues that the fit with the varying size Supreme Courts is just as good as with fixed size Supreme Courts.
DISCUSSION
(1) What is the probability that a President will make 2 or more appointments to the Supreme Court during a 4-year term? What is the probability a President makes no appointments during a 4-year term? Has this ever happened?
(2) Do you agree with Ulmer that there is no reason to limit the data to years with the Supreme Court is the same size?
(3) Obtain the data needed to update Ulmer's results to today and provide Tables that correspond to Ulmer's Tables 1 and 2. Add your Tables to this Chance News and make your data available to make it easier to update this in future years.
Contributed by Laurie Snell
Possible Cancer Cluster in Connecticut
Pratt Agrees to Increase Funding for Brain Cancer Study
The Associated Press
October 11, 2005
Stephen Singer
In a case that seems eerily familiar to the leukemia cancer cluster made famous by the book and movie, A Civil Action, researchers are trying to determine whether or not a cancer cluster exists among workers at Pratt & Whitney, a company that manufactures jet engines. Over one hundred former or current Pratt & Whitney employees have been identified as having a relatively rare type of fatal brain tumor known as glioblastoma multiforme, which strikes 3 people per 100,000 every year. Many of these workers have been exposed to metals and chemical substances, including TCE (trichloroethylene), which is an engine degreaser. TCE is the same carcinogenic chemical that was dumped in the 1960’s and 1970’s by the chemical company, WR Grace, in Woburn, MA, and that was found to have contaminated the drinking water in two of the town’s wells.
Prior to the recent announcement that the funding is being increased from $6 million dollars to $12 million dollars, this medical study was already being described as the largest occupational health study ever conducted. It covers 250,000 people who worked at any of the seven Connecticut Pratt & Whitney plants from 1952 to 2001. So far, at least 125 cases of brain cancer have been documented, according to the lawyer who represents dozens of individuals and family members.
The study falls under the auspices of the Connecticut Department of Public Health. However, due to the overwhelming cost and scope of the study, Pratt & Whitney is funding the project. Since Pratt is paying for the study, they chose the researchers, a team from the University of Pittsburgh. The project began three years ago, and the results are expected to be available between 2007 and 2009.
In the meantime, this case faces many of the obstacles common in the quest to establish whether or not a cancer cluster exists, such as proving causation, the fact that it can take a long time (sometimes 5-40 years) after exposure for true problems to show up, and finding the people who were exposed & convincing them to participate in the study. Additionally, there are legal difficulties for the families involved, including Connecticut’s statute of limitations laws, the length of time it takes investigators to reach a conclusion, the cost of litigation, and the “deep pockets” of a company like Pratt & Whitney.
This case study provides a current example of an ongoing investigation into a possible cancer cluster. Will it ultimately be found to be a coincidence cluster or proven to be a true cancer cluster? Does it make sense that the researchers are including in the study everyone who worked at Pratt over a fifty year period, even those who only worked there for a brief time period and those who were not directly exposed to toxic substances (i.e. office workers)? Could the researchers possibly have an unconscious bias in favor of the company that is funding their research? If the company doesn’t fund the research, then who should? Is there some other way to structure such investigations, so that the company involved pays for the study, but is not allowed to choose the researchers and directly fund them?
Further Reading
• “Rare Cancer Found in Workers”, The New York Times, March 10, 2002, Jane Gordon
• “Scientist: Pratt Commits to Cancer Study”, The Hartford Courant, May 19, 2005
• “Participation in Cancer Study at Pratt Lagging”, The Hartford Courant, October 12, 2005, Paul Marks
• The New Haven Advocate has had more than a dozen articles that explain the working conditions at Pratt & Whitney, and also chronicle the history & progression of this case. These articles have been investigated and written primarily by Carole Bass, Associate Editor. A few of the articles are listed below.
-“Worked to Death”, August 2, 2001, Carole Bass & Camille Jackson
-“Talking Cure”, October 28, 2004, Dave Goldberg
-“Time’s Not on Their Side”, January 20, 2005, Carole Bass
-“The Brains Behind the Brain”, October 20, 2005, Carole Bass
For the early New Haven Advocate articles (i.e., 2001-2002) on this subject, go to http://old.newhavenadvocate.com/workedtodeath/index.html
For the articles since 2002, go to
http://www.newhavenadvocate.com and search for Pratt & Whitney.
Submitted by Josephine Rodriguez.
A record powerball lottery jackpot
On Saturday Oct 22, 2005 the powerball lottery had a record powerball jackpot of 340 million dollars.
When you buy a ticket for this lottery, you specify five distinct numbers from 1 to 55, which we call basic numbers and one number from 1 to 42 called the bonus number. To win the Jackpot your 5 basic numbers and your bonus number must agree with a random choice of 5 basic numbers and one bonus number chosen by the lottery officials. The number of possible tickets is:
Thus if you buy a single ticket, the probability of having a winning jackpot ticket is
The powerball website and the media expresses this by saying that the odds that you have a winning jackpot ticket is 1 in 146,107,962.
Whenever there is a record jackpot, the news writers look for suggestions for other rare events that might be more familiar to its readers.
Our own favorite was suggested by Fred Hoppe: if you toss a coin 27 times your chance of getting 27 heads in a row is greater than your chance of having a winning jackpot ticket when you buy a single ticket. (<math> 2^{27} = 134217728</math> and so there are 134,217,728 possible sequences of heads and tails and only 1 has all heads.)
In an article for the Omaha World-Herald Oct 18, 2005 , Robynn Tysver writes:
You have a greater chance of getting killed by lightning than winning this week's 340 million Powerball jackpot. You also have a greater chance of drowning in a bathtub. The lifetime odds of getting killed by lightning are one in 56,439, and the lifetime odds of dying while in or falling into a bathtub are one in 10,582, according to the National Safety Council.
These are not even in the ballpark. The Minneapolis Star Tribune consulted mathematicians David Bressoud and Douglas Arnold who contributed the following:
According to Arnold you have a seven times better chance of becoming a saint. And Bressoud notes that you're six times more likely to get elected president of the United States. But they also provide the following more interesting estimates:
Bressoud: "If you buy 10 tickets a week, every week, it would take you 280,000 years before you could expect to win."
Arnold: "If you were to select a group of Powerball numbers every minute for 138 years, you would have about a 50 percent chance of picking the winning Powerball ticket."
It is not clear what Bressoud means by "expect to win". We can check this by a simple calculation (simple for Mathematica). Let q(n) be the probability that you if you buy n tickets you fail to get a winning jackpot ticket . Then:
<math> \left(\frac{146107961}{146107962}\right) ^n = q(n) </math> The average number of weeks in a year is 52.177457 so If you buy 10 tickets a week, every week for 280,000 years you will buy about 146,097,000 tickets. Using this number for n in our formula we find that you have about a 37% chance of not getting a winning lottery ticket or a 63% chance of getting one. We would not "expect to win" with a 63% chance of winning but others may. Using our formula for q(n) we find that if we buy 10 tickets a week, every week for 830,000 years you will have about a 95% chance of getting at least one winning lottery ticket which seems to us more appropriate for expecting to win.
Consider now Arnold's example. Our colleague Dana Williams found that you need to buy a ticket for about 101 million lottery offerings to have about a 50% chance of getting at least one winning lottery ticket. There are an average of 525,946.766 minutes in a year. Dividing 101 million by this number gives 192.035. Thus Arnold's 138 years should be 192 years.
We asked Professor Arnold about this and he replied:
That number, which was quoted in the Star Tribune was an off-the-top-of-my-head calculation made when the reporter called me on the phone with no warning. Right ballpark, but not very accurate. Later I redid the calculation carefully.
Arnold's computations were similar to ours but he used a greater accuracy for the number of independent lotteries you have to buy to have a 50% chance of choosing the jackpot numbers at least once. His answer to this was 101,274,322. Using this, Arnold's estimate for the number of years, buying a ticket every minute, to have a 50% chance of winning a jackpot is193 years instead of our estimate of 192 years.
Arnold said that he also estimated the expected value of a $1 ticket. To do this he needed an estimate for the number of tickets sold. He was told that a reasonable estimate was 106 million. In calculating the expected value of a $1 ticket he used the cash value of 164.4 million dollars instead of the annuity payout of 340 million dollars. He also took into account the possibility of having to share the jackpot. Doing this he got an expected value of exactly $1, just the price of the ticket. He commented that if he took into account the taxes the expected value would be more like 80 cents making even this lottery an unfavorable event.
The news writers also gave a lot of coverage of Senator Gregg (Chairman of the Senate Budget Committee) winning $853,492 for getting the second highest prize: getting the 5 basic numbers correct but note the bonus number. Normally the prize for getting this is $200,000 cash but Gregg was the beneficiary of recent change in the powerball lottery.
The powerball officials have found that increasing the size of the jackpot can increase the ticket sales because of the public's interest in large jackpots. They do this by increasing the number of possible basic numbers. When they started in 1992, to get the jackpot you had to get the 5 basic numbers chosen from 45 numbers and the 1 bonus number chosen from 45 number correct. Here are the changes so far
1992 5 of 45 1 of 45
1997 5 or 49 1 of 42
2002 5 of 53 1 of 42
2005 5 of 55 1 of 42
While the lottery officials want to have higher jackpots, they also do not want too long a period between jackpots. Normally the increase in the size of the lottery, when no one wins the jackpot, is determined by the amount taken in in this unsuccessful drawing. This was recently changed so that, when a new record jackpot occurs and no-one wins it, at most 25 million dollars are added to the Jackot until someone wins the jackpot. The money save by this is added to the second prize.
When the 340 million jackpot was won, 49 players had the five basic numbers correct but not the bonus numbers. For these winners the $200,000 prize had 653,492 added to their prize so they each won $853,492. If they chosen the Powerball play option for an extra $1 their $200,000 would have been multiplied by 2,3,4, or 5 with equal probabilities. Thus if Gregg had chosen the Powerball play option, and been lucky, he would have won 1,653,492 dollars that he could add to his 15 or so million dollars he has in stocks.
DISCUSSION
(1) Do you think it is fair to advertise the lottery prize as 340 million when the cash prize is only 164.4 million?
(2) What is the probability of winning the second prize?
(3) Assuming that the estimate 106 million for the number of tickets sold find the expected number of second prize winners.
Mammograms Validated as Key in Cancer Fight
The New York Times reported on October 27, 2005 on a new National Cancer Institute study that attributed a significant decrease in the death rate from breast cancer to mammogram screening tests. The study, published in the New England Journal of Medicine, found that from 28% to 65% of the sharp 24% decrease in breast cancer death rates from 1990 to 2000 was due to screening; the remainder was attributed to new drugs used to treat the disease.
Prior to this study, the value of mammogram screening had been disputed, for several reasons. In the general population, the test results in a large proportion number of false positives, about 90%. Thus, many women who are cancer-free are subjected to unnecessary procedures. Also, approximately 30% of cancers that are detected and treated would not have progressed significantly. Unfortunately, it isn't possible to distinguish between these so-called indolent cancers and those that become life-threatening, so it is routine practice to treat them all.
The new study suggests that the benefits of routine mammography are more certain than earlier believed, according to Don Berry, Chairman of the biostatistics department of M. D. Anderson Cancer Center, in Houston, Texas, and the lead author of the paper. He said that the new study for the first time properly separates the effects of therapy and screening. Nonetheless, he cautioned that mammography does pose risks, and recommended that women be counselled before screening.
Contributed by Bill Jefferys