Chance News 8: Difference between revisions
| Line 11: | Line 11: | ||
11 May 2005</div> | 11 May 2005</div> | ||
==The Poisson | ==The Poisson distribution and the Supreme Court== | ||
The Poisson | The Poisson distribution and the Supreme Court<br> | ||
''Journal of the American Statistical Association'' 31, no. 195 ,(1936), 376-80<br> | ''Journal of the American Statistical Association'' 31, no. 195 ,(1936), 376-80<br> | ||
W. Allen Wallis | W. Allen Wallis | ||
Supreme Court Appointments as a Poisson | Supreme Court Appointments as a Poisson distribution<br> | ||
''American Journal of Political Science'', 26, No.1, February 1982<br> | ''American Journal of Political Science'', 26, No.1, February 1982<br> | ||
S. Sidney Ulmer | S. Sidney Ulmer | ||
| Line 22: | Line 22: | ||
This is not current news, but since Supreme Court appointments are in the news we felt that these articles might make an interesting class discussion. | This is not current news, but since Supreme Court appointments are in the news we felt that these articles might make an interesting class discussion. | ||
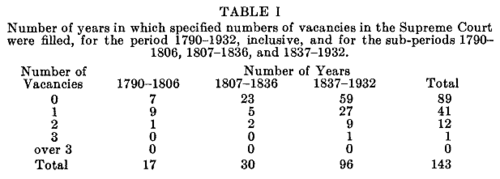
In 1936 statistician Allen Wallis suggested that the number of Supreme Court | In 1936 statistician Allen Wallis suggested that a Poisson distribution can approximate the number of U.S. Supreme Court appointments in a given year. In his Table 1 Wallis provided the number appointed in each year over the intervals with different numbers of Justices on the Supreme Court: 1790-1806 (6) 1807-1836 (7), and 1837-1932 (9). Actually there were 10 in the years 1963 to 1967 which Wallis called "negligible exceptions." | ||
[[Image:wallis1.png|500px|center]] | [[Image:wallis1.png|500px|center]] | ||
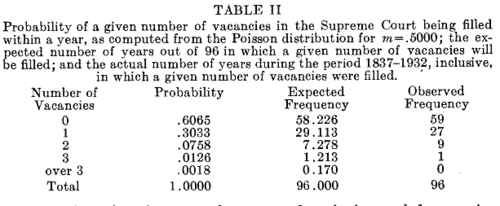
Wallis then restricts himself to the years 1837-1932 and finds that the number of court | Wallis then restricts himself to the years 1837-1932 and finds that a Poisson distribution with mean .5 can approximate the number of court appointments in a year. His results are shown in his Figure II: | ||
[[Image:wallis2.png|500px|center]] | [[Image:wallis2.png|500px|center]] | ||
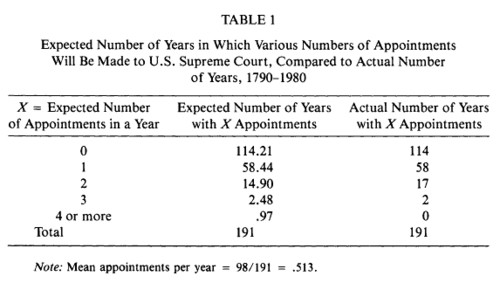
In his article, Sidney Ulmer updated the data to 1980 and also includes data from the early Supreme Courts with 6 and 7 members. Thus his data covers the years 1790--1980. His Table 1 compares the actual number of | In his article, Sidney Ulmer updated the data to 1980 and also includes data from the early Supreme Courts with 6 and 7 members. Thus his data covers the years 1790--1980. His Table 1 compares the actual number of appointments in a year with the expected number of appointments assuming a Poisson distribution with mean 5.13. | ||
[[Image:ulmer1.png|500px|center]] | [[Image:ulmer1.png|500px|center]] | ||
| Line 38: | Line 38: | ||
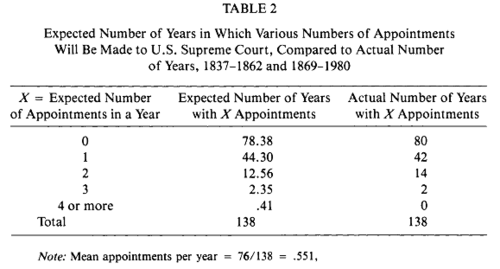
[[Image:ulmer2.png|500px|center]] | [[Image:ulmer2.png|500px|center]] | ||
Testing the goodness of | Testing the goodness of fits, Ulmer argues that the fit with the varying size Supreme Courts is just as good as with fixed size Supreme Courts. | ||
===DISCUSSION=== | ===DISCUSSION=== | ||
(1) What is the | (1) What is the probability that a President will make 2 or more appointments to the Supreme Court during a 4-year term? What is the probability a President makes no appointments during a 4-year term? Has this ever happened? | ||
(2) Do you agree with | (2) Do you agree with Ulmer that there is no reason to limit the data to years with the Supreme Court is the same size? | ||
(3) Obtain the data needed to update | (3) Obtain the data needed to update Ulmer's results to 1980 and provide Tables to correspond to Ulmer's Tables 1 and 2. Add your data Tables to this Chance News. | ||
(1) Find the probability that a President who serves four years will make 2 or more Supreme Court appointments. | |||
(2) Obtain the data from 1790 to 2005 and make tables corresponding to the Ulmer's Tables 1 and 2. Add the tables to this article and also include the data to make it easier to update these results in future years. | |||
==DISCUSSION== | ==DISCUSSION== | ||
Revision as of 19:52, 23 October 2005
Oct 15 to Oct 30
Quotation
One more fagot of these adamantine bandages, is, the new science of Statistics. It is a rule, that the most casual and extraordinary events -- if the basis of population is broad enough -- become matter of fixed calculation. It would not be safe to say when a captain like Bonaparte, a singer like Jenny Lind, or a navigator like Bowditch, would be born in Boston: but, on a population of twenty or two hundred millions, something like accuracy may be had. Ralph Waldo Emerson Fate
Forsooth
Here's another Forsooth from the October issue of RSS News.
Your'e more likely to die in a fire in Strathclyde than anywhere else in the country
11 May 2005
The Poisson distribution and the Supreme Court
The Poisson distribution and the Supreme Court
Journal of the American Statistical Association 31, no. 195 ,(1936), 376-80
W. Allen Wallis
Supreme Court Appointments as a Poisson distribution
American Journal of Political Science, 26, No.1, February 1982
S. Sidney Ulmer
This is not current news, but since Supreme Court appointments are in the news we felt that these articles might make an interesting class discussion.
In 1936 statistician Allen Wallis suggested that a Poisson distribution can approximate the number of U.S. Supreme Court appointments in a given year. In his Table 1 Wallis provided the number appointed in each year over the intervals with different numbers of Justices on the Supreme Court: 1790-1806 (6) 1807-1836 (7), and 1837-1932 (9). Actually there were 10 in the years 1963 to 1967 which Wallis called "negligible exceptions."
Wallis then restricts himself to the years 1837-1932 and finds that a Poisson distribution with mean .5 can approximate the number of court appointments in a year. His results are shown in his Figure II:
In his article, Sidney Ulmer updated the data to 1980 and also includes data from the early Supreme Courts with 6 and 7 members. Thus his data covers the years 1790--1980. His Table 1 compares the actual number of appointments in a year with the expected number of appointments assuming a Poisson distribution with mean 5.13.
In his Table II Ulmer limits the data to the 9 member Supreme Courts: 1837 to 1862 and 1869-1980).
Testing the goodness of fits, Ulmer argues that the fit with the varying size Supreme Courts is just as good as with fixed size Supreme Courts.
DISCUSSION
(1) What is the probability that a President will make 2 or more appointments to the Supreme Court during a 4-year term? What is the probability a President makes no appointments during a 4-year term? Has this ever happened?
(2) Do you agree with Ulmer that there is no reason to limit the data to years with the Supreme Court is the same size?
(3) Obtain the data needed to update Ulmer's results to 1980 and provide Tables to correspond to Ulmer's Tables 1 and 2. Add your data Tables to this Chance News.
(1) Find the probability that a President who serves four years will make 2 or more Supreme Court appointments.
(2) Obtain the data from 1790 to 2005 and make tables corresponding to the Ulmer's Tables 1 and 2. Add the tables to this article and also include the data to make it easier to update these results in future years.
DISCUSSION
(1) Find the probability that a president who serves four years will make 2 or more Supreme Court appointments.
(2) Obtain the data from 1790 to 2005 and make tables corresponding to the Ulman's Tables 1 and 2. Add the tables to this article and also include the data to make it easier to update these results in future years.
Item2
To be added.