Chance News 106: Difference between revisions
m (→Forsooth) |
|||
| Line 42: | Line 42: | ||
---- | ---- | ||
“Saves 88% more water than a one-gallon urinal.” | “Saves 88% more water than a one-gallon urinal.” | ||
<div align=right>--in: washrooms throughout | <div align=right>--in: washrooms throughout Washington State Convention Center, JSM 2015</div> | ||
We are not the first to notice. This image | We are not the first to notice. This image | ||
Revision as of 19:43, 19 August 2015
Quotations
“[A]ccording to preliminary 2014 statistics from the National Transportation Safety Board, there were no fatal accidents on U.S. commercial airline flights .... But 419 people died in general aviation crashes, a figure that inflates the average American’s odds of dying in a plane crash, even though most of us don’t travel in small, private planes.”
Submitted by Margaret Cibes
Forsooth
You really are old as you feel
"Some of the subjects 'aged physiologically not at all [while] at the other extreme there were folks aging two to three times as much.'"
Submitted by Chris Andrews
See amazing comments here, as bloggers try to figure out the arithmetic.
Submitted by Margaret Cibes at the suggestion of Sarah Bedichek
Most Common Movie Title: Alice in Wonderland
1. Average IMDb Rating: 6.0376514
Most Common IMDb Rating: 7.2
Standard Deviation of IMDb Rating: 1.2209091978981308
2. Average Movie Length: 100 minutes
Most Common Movie Length: 90 minutes
Standard Deviation: 31.94308331064542
3. Average IMDb User Votes: 27,627
Most Common Number of User Votes: 5
Standard Deviation: 75824.97716775243 ....
5. Average Movie Year: 1984
Most Common Movie Year: 2004
Standard Deviation: 25.087728495416965
Submitted by Margaret Cibes at the suggestion of James Greenwood
“Saves 88% more water than a one-gallon urinal.”
We are not the first to notice. This image
appears with commentary at What a urinal can teach us about statistics, "Armchair MBA' blog, 15 December 2014
Submitted by Bill Peterson
More on the hot hand
In Chance News 105, the last item was titled Does selection bias explain the hot hand?. It described how in their July 6 article, Miller and Sanjurjo assert that to determine the probability of a heads following a heads in a fixed sequence, you may calculate the proportion of times a head is followed by a head for each possible sequence and then compute the average proportion, giving each sequence an equal weighting on the grounds that each possible sequence is equally likely to occur. I agree that each possible sequence is equally likely to occur. But I assert that it is illegitimate to weight each sequence equally because some sequences have more chances for a head to follow a second head than others.
Let us assume, as Miller and Sanjurjo do, that we are considering the 14 possible sequences of four flips containing at least one head in the first three flips. A head is followed by another head in only one of the six sequences (see below) that contain only one head that could be followed by another, making the probability of a head being followed by another 1/6 for this set of six sequences.
TTHT Heads follows heads 0 time THTT Heads follows heads 0 times HTTT Heads follows heads 0 times TTHH Heads follows heads 1 time THTH Heads follows heads 0 times HTTH Heads follows heads 0 times
A head is followed by another head six out of 12 times in the six sequences (see below) that contain two heads that could be followed by another head, making the probability of a head being followed by another 6/12 = 1/2 for this set of six sequences.
THHT Heads follows heads 1 time HTHT Heads follows heads 0 times HHTT Heads follows heads 1 time THHH Heads follows heads 2 times HTHH Heads follows heads 1 time HHTH Heads follows heads 1 time
A head is followed by another head five out of six times in the two sequences (see below) that contain three heads that could be followed by another head, making the probability of a head being followed by another 5/6 this set of two sequences.
HHHT Heads follows heads 2 times HHHH Heads follows heads 3 times
An unweighted average of the 14 sequences gives
- [(6 × 1/6) + (6 × 1/2) + (2 × 5/6)] / 14 = [17/3] / 14 = 0.405,
which is what Miller and Sanjurjo report. A weighted average of the 14 sequences gives
- [(1)(6 × 1/6) + (2)(6 × 1/2) + (3)(2 × 5/6)] / [(1×6) + (2 × 6) + (3 × 2)]
- = [1 + 6 + 5] / [6 + 12 + 6] = 12/24 = 0.50.
Using an unweighted average instead of a weighted average is the pattern of reasoning underlying the statistical artifact known as Simpson’s paradox. And as is the case with Simpson’s paradox, it leads to faulty conclusions about how the world works.
Submitted by Jeff Eiseman, University of Massachusetts
Predicting GOP debate participants
Ethan Brown posted this following link on the Isolated Statisticians list:
- The first G.O.P. debate: Who’s in, who’s out and the role of chance
- by Kevin Quealy and Amanda Cox , "Upshot" blog New York Times, 21 July 2015
Because of the large number of declared candidates (16 and growing at the time of the article), Fox News has limited participation in its August 6 debate to those who meet the following criterion
Must place in the top 10 of an average of the five most recent national polls, as recognized by FOX News leading up to August 4th at 5 PM/ET. Such polling must be conducted by major, nationally recognized organizations that use standard methodological techniques.
But of course, polls are subject to sampling error. The NYT article uses simulation to illustrate how this could affect participation. Supposing the latest polling averages represent the "correct" values, they simulate 5 additional polls (as Ethan noted, this is a bootstrapping approach). The results demonstrate that this can affect who's in and who's out, as well as the location on the stage for those who do make the cut.
The Washington Post maintains a State of the debate widget that updates the current top 10 based on the most recent polling results. For July 30 we see

Note that the top figure is not a bar chart; it represents the positions of the candidates on the stage.
Updates
Who gets into the Republican debate: Rounding could decide
by Kevin Quealy, "Upshot" blog, New York Times, 3 August 2015
The article points out that Fox has not specified if or how it will round the polling figures. For example, the 9th, 10th and 11th places as of July 30 (shown in the display above) were Chris Christie (3.3%), John Kasich (2.7%) and Rick Perry (2.3%). These are all within a one percentage point range, but Christie and Kasich currently in in the top 10, but not Perry. More recent data presented in the present article have Christie and Kasich at 3.8% and Perry at 2.8%, a tie figures are rounded to 3%.
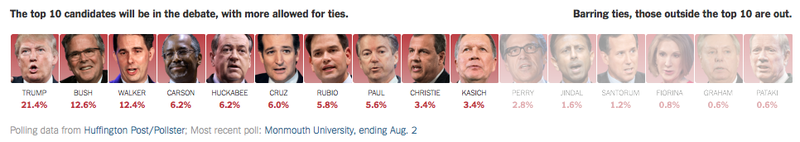
Quealy wonders if Fox might then consider having 11 debaters?
Final update: The NYT reported on August 4 that John Kasich Is In, Rick Perry Is out in first Republican debate. The article quotes a statement from Fox News:
Each poll has a different margin of error, and averaging requires a distinct test of statistical significance. Given the over 2,400 interviews contained within the five polls, from a purely statistical perspective it is at least 90 percent likely that the 10th place Kasich is ahead of the 11th place Perry.”
Discussion
How do you think Fox arrived at their "90 percent likely" statement?
Evaluating health studies
We all know that as general as statistics is, particular subject matter knowledge and familiarity are all important. Here is a useful link for understanding and critically evaluating health news:
- NPR’s On the Media with a skeptic’s guide to health news/diet fads
- by Gary Schwitzer, HealthNewsReviews.org, 31 July 2015
From which we find a consumer’s handbook for evaluating a health study:

The On the Media web site also contains a lengthy radio interview dealing with most of the above items.
Submitted by Paul Alper
Cautions on hypothesis testing
Scientific method: Statistical errors
by Regina Nuzzo, Nature News, 12 February 2014
The subtitle is "P values, the 'gold standard' of statistical validity, are not as reliable as many scientists assume." We read:
P values have always had critics. In their almost nine decades of existence, they have been likened to mosquitoes (annoying and impossible to swat away), the emperor's new clothes (fraught with obvious problems that everyone ignores) and the tool of a “sterile intellectual rake” who ravishes science but leaves it with no progeny[1]. One researcher suggested rechristening the methodology “statistical hypothesis inference testing”[2], presumably for the acronym it would yield.
Later on we have:
But while the rivals feuded — Neyman called some of Fisher's work mathematically “worse than useless”; Fisher called Neyman's approach “childish” and “horrifying [for] intellectual freedom in the west” — other researchers lost patience and began to write statistics manuals for working scientists. And because many of the authors were non-statisticians without a thorough understanding of either approach, they created a hybrid system that crammed Fisher's easy-to-calculate P value into Neyman and Pearson's reassuringly rigorous rule-based system. This is when a P value of 0.05 became enshrined as 'statistically significant', for example. “The P value was never meant to be used the way it's used today,” says [Steven] Goodman.
I have always bemoaned the conflation of exploratory and confirmatory:
Such practices have the effect of turning discoveries from exploratory studies — which should be treated with scepticism — into what look like sound confirmations but vanish on replication.
Submitted by Paul Alper

