Sandbox

Gini coefficient for air travel

Peter Doyle sent a link to the following:

Modern flying: a triumph in socialism

Economist, 4 December 2014

The article poses the question "Is a commercial jumbo jet a more or a less equal place to be than America?" In a blog post, SUNY Albany sociologist Beth Berman suggested computing the Gini coefficient for the amount of space allocated to higher class passengers relative to their numbers. According to Berman

...in today’s standard U.S. domestic configuration, the 12% of people in first class use about 25% of the passenger space, the 51 people in Economy Plus use another 30%, leaving the sardines—the other 157 people—with 45%. That gives us a Gini index of about 16.

The article reports that the US Gini coefficient for income is 41, making the airline's allocation of space more egalitarian. Even the new Boeing 777, whose luxury accommodations include horizontal air beds, has a coefficient of only 25.

In a related blog post entitled Fly Air Gini, Duke sociologist Kieran Healy produced graphics depicting what things would look where space was allocated to match (income) Gini coefficient.

More on Gini

The analysis received news coverage elsewhere, for example:

How airline seating reflects income inequality

by Michael Hiltzik, Los Angeles Times, 2 December 2014

Some math doodles

<math>P \left({A_1 \cup A_2}\right) = P\left({A_1}\right) + P\left({A_2}\right) -P \left({A_1 \cap A_2}\right)</math>

Accidental insights

My collective understanding of Power Laws would fit beneath the shallow end of the long tail. Curiosity, however, easily fills the fat end. I long have been intrigued by the concept and the surprisingly common appearance of power laws in varied natural, social and organizational dynamics. But, am I just seeing a statistical novelty or is there meaning and utility in Power Law relationships? Here’s a case in point.

While carrying a pair of 10 lb. hand weights one, by chance, slipped from my grasp and fell onto a piece of ceramic tile I had left on the carpeted floor. The fractured tile was inconsequential, meant for the trash.

As I stared, slightly annoyed, at the mess, a favorite maxim of the Greek philosopher, Epictetus, came to mind: “On the occasion of every accident that befalls you, turn to yourself and ask what power you have to put it to use.” Could this array of large and small polygons form a Power Law? With curiosity piqued, I collected all the fragments and measured the area of each piece.

Piece	Sq. Inches	% of Total
1	43.25	31.9%
2	35.25	26.0%
3	23.25	17.2%
4	14.10	10.4%
5	7.10	5.2%
6	4.70	3.5%
7	3.60	2.7%
8	3.03	2.2%
9	0.66	0.5%
10	0.61	0.5%

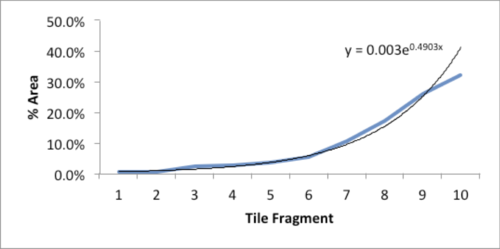
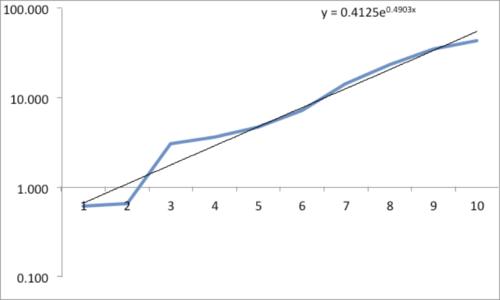
The data and plot look like a Power Law distribution. The first plot is an exponential fit of percent total area. The second plot is same data on a log normal format. Clue: Ok, data fits a straight line. I found myself again in the shallow end of the knowledge curve. Does the data reflect a Power Law or something else, and if it does what does it reflect? What insights can I gain from this accident? Favorite maxims of Epictetus and Pasteur echoed in my head: “On the occasion of every accident that befalls you, remember to turn to yourself and inquire what power you have to turn it to use” and “Chance favors only the prepared mind.”

My “prepared” mind searched for answers, leading me down varied learning paths. Tapping the power of networks, I dropped a note to Chance News editor Bill Peterson. His quick web search surfaced a story from Nature News on research by Hans Herrmann, et. al. Shattered eggs reveal secrets of explosions. As described there, researchers have found power-law relationships for the fragments produced by shattering a pane of glass or breaking a solid object, such as a stone. Seems there is a science underpinning how things break and explode; potentially useful in Forensic reconstructions. Bill also provided a link to a vignette from CRAN describing a maximum likelihood procedure for fitting a Power Law relationship. I am now learning my way through that.

Submitted by William Montante

Cancer and luck

Cancer’s random assault
By Denise Grady, New York Times, 5 January, 2015

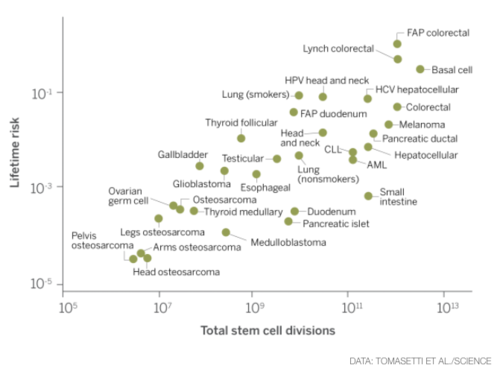
The article concerns a recent research paper, Variation in cancer risk among tissues can be explained by the number of stem cell divisions (Science 2 January 2015). From the abstract

Here, we show that the lifetime risk of cancers of many different types is strongly correlated (0.81) with the total number of divisions of the normal self-renewing cells maintaining that tissue’s homeostasis. These results suggest that only a third of the variation in cancer risk among tissues is attributable to environmental factors or inherited predispositions.

News coverage has created controversy by summarizing the findings in more colloquial terms, similar to this from the NYT article:

Random mutations may account for two-thirds of the risk of getting many types of cancer, leaving the usual suspects — heredity and environmental factors — to account for only one-third, say the authors, Cristian Tomasetti and Dr. Bert Vogelstein, of Johns Hopkins University School of Medicine.

Of course, saying that two-thirds of the variation among cancer types is explained the rate of cell division is not the same thing as saying that two-thirds of risk of a particular cancer is due can be accounted for by chance.

Bad luck and cancer: A science reporter’s reflections on a controversial story
by Science Insider,

We can now see where the two-thirds comes from: if <math>r = 0.81</math> then <math>R^2=0.81</math>.