Sandbox: Difference between revisions

From ChanceWiki
Jump to navigation Jump to search
 
Line 1: Line 1:
==[Poker Showdown Between Luck and Skill==
[http://blogs.wsj.com/numbersguy/poker-showdown-between-luck-and-skill-649/ The Numbers Guy]<br>
March 27, 2009


The Wall Street Journal has a number  of Blogs. One of these is call "The Numbers Guy"  and maintaigned by
Carl Bialik who writes the way numbers are used, and abused. Many of his articles occure in the Wall Street Journal. 


In this article Bialik writes: Is Texas Hold ‘Em poker more a game of chance or of skill? That question has figured in several legal tests of playing the card game for money: Games of chance are considered gambling under U.S. law. Now a major poker Web site has sponsored a study it claims demonstrates that it takes skill to win — which would help the site’s legal standing. But several poker experts question that claim.</blockquote>
==Forsooth==


We discussed this problem in previus issues of Chance news [http://chance.dartmouth.edu/chancewiki/index.php/Chance_News_29#Is_Poker_predominantly_skill_or_luck.3F here] and [http://chance.dartmouth.edu/chancewiki/index.php/Chance_News_30#Is_poker_predomately_a_game_of_skill_or_luck.3F_revisited here]
==Quotations==
“We know that people tend to overestimate the frequency of well-publicized, spectacular
events compared with more commonplace ones; this is a well-understood phenomenon in
the literature of risk assessment and leads to the truism that when statistics plays folklore,
folklore always wins in a rout.”
<div align=right>-- Donald Kennedy (former president of Stanford University), ''Academic Duty'', Harvard University Press, 1997, p.17</div>


Texas Holdem like other poker games starts with the the players being delt a number of cards.  Then the players put must put a prescribed amount of money in the "pot".  Then in a number of stages the players  make bets which other players must match or drop out of the game.  When there are no more bets there is a "showdown" among the players still in the game. The player with the best hand wins the money in the pot or if their are ties the money is shared. You can read the rules for playing Texas Holdem here
----
   
Bailick writes: "PokerStars paid Cigital, a software consulting firm, to analyze 103,273,484 hands played on the site last December, for real money — usually at least $1 blind bets.  Three quarters of the hands analyzed ended without a showdown, meaning that the winner never had to show his or her cards — everyone else eventually folded during the rounds of betting. And half the time that hands did end in a showdown, a player who would have won had already folded.


Paco Hope, technical manager at Cigital and co-author of the study, argues that the paucity of showdowns shows poker is a game of skill: The winner could have won by making identical bets no matter which cards he or she had drawn. “Most people think, you get your cards, and the best hand wins,” Hope said. He added, “Whether or not you go to a showdown is determined by the decisions you make, which are determined entirely by your skill.”
"Using scientific language and measurement doesn’t prevent a researcher from conducting flawed experiments and drawing wrong conclusions — especially when they confirm preconceptions."


Bailick writes: "Who folds is determined to a huge degree by the value of the cards! Peter Winkler, a Dartmouth College mathematician who has studied games of skill and chance, said in an email. The player who picks up AA [two aces] and stays in while the rest fold is the lucky one; the player who picks up 32 [a three and a two] and folds before the 332 [three, three, two] flop comes down is the unlucky one. That the AA player wins with an ultimately inferior hand does not prove poker is a game of skill. If anything, it shows the opposite: an unskillful player holding the 32 hole cards might have stayed in.
<div align=right>-- Blaise Agüera y Arcas, Margaret Mitchell and Alexander Todoorov, quoted in: The racist history behind facial recognition, ''New York Times'', 10 July 2019</div>


Hope counters that skill dominates luck in decision-making: “The same information is available to all players (the values of the cards), but it is skill in interpreting that information — not the presence of that information — that determines whether a player folds.
==In progress==
[https://www.nytimes.com/2018/11/07/magazine/placebo-effect-medicine.html What if the Placebo Effect Isn’t a Trick?]<br>
by Gary Greenberg, ''New York Times Magazine'', 7 November 2018


As for the failure to track individual players, Hope argues that it doesn’t matter whether skillful competitors are identified. “I don’t care who won or why they won,” he said. “What I care about is the decisions they made. The fact they decided to fold indicates it was decisions that determined the hand.
[https://www.nytimes.com/2019/07/17/opinion/pretrial-ai.html The Problems With Risk Assessment Tools]<br>
by Chelsea Barabas, Karthik Dinakar and Colin Doyle, ''New York Times'', 17 July 2019


Like other Blogs Bailick invites readers to comment on these arguments.  Particularly interesting comments were made by Patrick Fleming.  He writes:
==Hurricane Maria deaths==
Laura Kapitula sent the following to the Isolated Statisticians e-mail list:


Your analysis of the Cigital study is much too simplistic. Mr. Hope is not saying that because the majority of poker hands are resolved without a showdown that ALONE means poker is a game of skill. What is shows is that the vast majority of poker hands are determined by the way people play the cards, not the actual deal of the cards. IF it is also true that the way people play the cards is an exercise of skill, THEN it follows that the exercise of skill is the predominant factor in determining the outcome of poker.
:[Why counting casualties after a hurricane is so hard]<br>
First, Isn’t knowing that your AA hand is likely to be the winner in and of itself an application of knowledge and therefore a skill? But even if you want more than that to qualify as “skill,” there is more, indeed much more.
:by Jo Craven McGinty, Wall Street Journal, 7 September 2018


Your statement “For one thing, players’ decisions are determined by the cards they draw, which is entirely a matter of luck.” is patently false, and I am surprised you of all folks made it. I know from previous blogs that you are familiar with the rules of poker. A player’s decision is NEVER “determined” by the cards they hold (except for a few very minor points, like who makes the first mandatory bet in some version of stud poker). The cards a player holds may influence his decision, indeed sometimes they may be the biggest factor in his decision, but they never (except as noted above) DETERMINE his decision. A player in hold-em is just as free to raise with 3-2 as he is to fold 3-2. What he actually decides to do with that 3-2 will depend on a number of other factors. If he is a skilled player, he may realize that the other players are “scared” of him. He may raise knowing that they will think he has much better cards. If he does that and the other players fold, he wins. His actual cards had nothing to do with his win in that situation.
The article is subtitled: Indirect deaths—such as those caused by gaps in medication—can occur months after a storm, complicating tallies
Laura noted that
:[https://www.washingtonpost.com/news/fact-checker/wp/2018/06/02/did-4645-people-die-in-hurricane-maria-nope/?utm_term=.0a5e6e48bf11 Did 4,645 people die in Hurricane Maria? Nope.]<br>
:by Glenn Kessler, ''Washington Post'', 1 June 2018


Likewise, the comment by Mr. Winkler that ““Who folds is determined to a huge degree by the value of the cards!” shows a lack of understanding of poker. I doubt Mr. Winkler has played much poker. A player who bases his decisions solely or “to a huge degree” on what cards they hold is at best a beginning player, and will not be a successful player until they start to consider other factors before making their decisions. What cards you hold, as any poker pro will tell you, is only the beginning of the thought process. As Kenny Rogers sang, every hands a winner, and every hands a loser. Most of the time which one your 2 cards will turn out to be depends on many, many other factors.
The source of the 4645 figure is a [https://www.nejm.org/doi/full/10.1056/NEJMsa1803972 NEJM article]. Point estimate, the 95% confidence interval ran from 793 to 8498.


This comments section is not the place I want to begin a discussion of all of the factors that go into deciding how to play a poker hand, whole books have been written on the subject, so I will just list a view: your image as a player, your position at the table, the size of the bet, the size of your bankroll, the styles of the other players, physical tells of the other players, and mathematical probability. Analyzing and applying all of these and other concerns, plus the actual cards you hold, is a very complex thought process. It is a learned skill, and a skill that can be improved. And that proves the second half of the equation, and thats why this study HELPS to prove that the results in poker are MOSTLY the product of player skill.
President Trump has asserted that the actual number is
And please note my use of the word “mostly,” No one denies that chance determines some results in poker. When you raise “all in” early with A-A and an opponent calls with 9-10 cause he mistakenly thinks you are bluffing, and 2 remaining 10s are dealt (with no ace), chance has determined that outcome for the most part.
[https://twitter.com/realDonaldTrump/status/1040217897703026689 6 to 18].
The ''Post'' article notes that Puerto Rican official had asked researchers at George Washington University to do an estimate of the death toll.  That work is not complete.
[https://prstudy.publichealth.gwu.edu/ George Washington University study]


But the question is not whether chance determines EVERY outcome in poker, nor whether skill determines EVERY outcome. The question is which is determining the MAJORITY of outcomes. Since in the majority of outcomes the cards, as shown by this study, are not even consulted, that is strong proof that the majority of outcomes fall into the skilled category. And that becomes conclusive proof when one looks at the actual decision making process required in MOST poker situations, and realizes that it involves complex analysis frequently unrelated to the actual cards held.
:[https://fivethirtyeight.com/features/we-still-dont-know-how-many-people-died-because-of-katrina/?ex_cid=538twitter We sttill don’t know how many people died because of Katrina]<br>
:by Carl Bialik, FiveThirtyEight, 26 August 2015


And one final point, to the expert who asserts a better test would be to show that skilled players win more often, has he ever heard of Doyle Brunson? 50 years of being a successful poker player must have more to do with skill than with Doyle just being the luckiest person on the planet, dont you think?
----
In sum, the Cigital study is only one piece of a larger analytic framework, but it is an important piece. And when all of the pieces are put together, the evidence that poker is a game of PREDOMINANTLY skill is, as the judge in South Carolina recently stated, “overwhelming.”
[https://www.nytimes.com/2018/09/11/climate/hurricane-evacuation-path-forecasts.html These 3 Hurricane Misconceptions Can Be Dangerous. Scientists Want to Clear Them Up.]<br>
[https://journals.ametsoc.org/doi/abs/10.1175/BAMS-88-5-651 Misinterpretations of the “Cone of Uncertainty” in Florida during the 2004 Hurricane Season]<br>
[https://www.nhc.noaa.gov/aboutcone.shtml Definition of the NHC Track Forecast Cone]
----
[https://www.popsci.com/moderate-drinking-benefits-risks Remember when a glass of wine a day was good for you? Here's why that changed.]
''Popular Science'', 10 September 2018
----
[https://www.economist.com/united-states/2018/08/30/googling-the-news Googling the news]<br>
''Economist'', 1 September 2018


Thank you,
Patrick Fleming
[https://www.cnbc.com/2018/09/17/google-tests-changes-to-its-search-algorithm-how-search-works.html We sat in on an internal Google meeting where they talked about changing the search algorithm — here's what we learned]
----
[http://www.wyso.org/post/stats-stories-reading-writing-and-risk-literacy Reading , Writing and Risk Literacy]


Full disclosure - I am the Poker Players Alliance Litigation Support Director and helped craft the argument that has been presented in the courts
[http://www.riskliteracy.org/]
-----
[https://twitter.com/i/moments/1025000711539572737?cn=ZmxleGlibGVfcmVjc18y&refsrc=email Today is the deadliest day of the year for car wrecks in the U.S.]
 
==Some math doodles==
<math>P \left({A_1 \cup A_2}\right) = P\left({A_1}\right) + P\left({A_2}\right) -P \left({A_1 \cap A_2}\right)</math>
 
<math>P(E)  = {n \choose k} p^k (1-p)^{ n-k}</math>
 
<math>\hat{p}(H|H)</math>
 
<math>\hat{p}(H|HH)</math>
 
==Accidental insights==
 
My collective understanding of Power Laws would fit beneath the shallow end of the long tail. Curiosity, however, easily fills the fat end.  I long have been intrigued by the concept and the surprisingly common appearance of power laws in varied natural, social and organizational dynamics.  But, am I just seeing a statistical novelty or is there meaning and utility in Power Law relationships? Here’s a case in point.
 
While carrying a pair of 10 lb. hand weights one, by chance, slipped from my grasp and fell onto a piece of ceramic tile I had left on the carpeted floor. The fractured tile was inconsequential, meant for the trash.
<center>[[File:BrokenTile.jpg | 400px]]</center>
As I stared, slightly annoyed, at the mess, a favorite maxim of the Greek philosopher, Epictetus, came to mind: “On the occasion of every accident that befalls you, turn to yourself and ask what power you have to put it to use.”  Could this array of large and small polygons form a Power Law? With curiosity piqued, I collected all the fragments and measured the area of each piece.
 
<center>
{| class="wikitable"
|-
! Piece !! Sq. Inches !! % of Total
|-
| 1 || 43.25 || 31.9%
|-
| 2 || 35.25 ||26.0%
|-
|  3 || 23.25 || 17.2%
|-
| 4 || 14.10 || 10.4%
|-
| 5 || 7.10 || 5.2%
|-
| 6 || 4.70 || 3.5%
|-
| 7 || 3.60 || 2.7%
|-
| 8 || 3.03 || 2.2%
|-
| 9 || 0.66 || 0.5%
|-
| 10 || 0.61 || 0.5%
|}
</center>
<center>[[File:Montante_plot1.png | 500px]]</center>
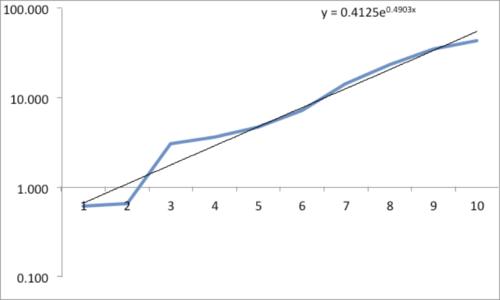
The data and plot look like a Power Law distribution. The first plot is an exponential fit of percent total area. The second plot is same data on a log normal format. Clue: Ok, data fits a straight line.  I found myself again in the shallow end of the knowledge curve. Does the data reflect a Power Law or something else, and if it does what does it reflect?  What insights can I gain from this accident? Favorite maxims of Epictetus and Pasteur echoed in my head:
“On the occasion of every accident that befalls you, remember to turn to yourself and inquire what power you have to turn it to use” and “Chance favors only the prepared mind.”
 
<center>[[File:Montante_plot2.png | 500px]]</center>
My “prepared” mind searched for answers, leading me down varied learning paths. Tapping the power of networks, I dropped a note to Chance News editor Bill Peterson. His quick web search surfaced a story from ''Nature News'' on research by Hans Herrmann, et. al. [http://www.nature.com/news/2004/040227/full/news040223-11.html Shattered eggs reveal secrets of explosions].  As described there, researchers have found power-law relationships for the fragments produced by shattering a pane of glass or breaking a solid object, such as a stone. Seems there is a science underpinning how things break and explode; potentially useful in Forensic reconstructions.
Bill also provided a link to [http://cran.r-project.org/web/packages/poweRlaw/vignettes/poweRlaw.pdf a vignette from CRAN] describing a maximum likelihood procedure for fitting a Power Law relationship. I am now learning my way through that.
 
Submitted by William Montante
 
----

Latest revision as of 20:58, 17 July 2019


Forsooth

Quotations

“We know that people tend to overestimate the frequency of well-publicized, spectacular events compared with more commonplace ones; this is a well-understood phenomenon in the literature of risk assessment and leads to the truism that when statistics plays folklore, folklore always wins in a rout.”

-- Donald Kennedy (former president of Stanford University), Academic Duty, Harvard University Press, 1997, p.17

"Using scientific language and measurement doesn’t prevent a researcher from conducting flawed experiments and drawing wrong conclusions — especially when they confirm preconceptions."

-- Blaise Agüera y Arcas, Margaret Mitchell and Alexander Todoorov, quoted in: The racist history behind facial recognition, New York Times, 10 July 2019

In progress

What if the Placebo Effect Isn’t a Trick?
by Gary Greenberg, New York Times Magazine, 7 November 2018

The Problems With Risk Assessment Tools
by Chelsea Barabas, Karthik Dinakar and Colin Doyle, New York Times, 17 July 2019

Hurricane Maria deaths

Laura Kapitula sent the following to the Isolated Statisticians e-mail list:

[Why counting casualties after a hurricane is so hard]
by Jo Craven McGinty, Wall Street Journal, 7 September 2018

The article is subtitled: Indirect deaths—such as those caused by gaps in medication—can occur months after a storm, complicating tallies

Laura noted that

Did 4,645 people die in Hurricane Maria? Nope.
by Glenn Kessler, Washington Post, 1 June 2018

The source of the 4645 figure is a NEJM article. Point estimate, the 95% confidence interval ran from 793 to 8498.

President Trump has asserted that the actual number is 6 to 18. The Post article notes that Puerto Rican official had asked researchers at George Washington University to do an estimate of the death toll. That work is not complete. George Washington University study

We sttill don’t know how many people died because of Katrina
by Carl Bialik, FiveThirtyEight, 26 August 2015

These 3 Hurricane Misconceptions Can Be Dangerous. Scientists Want to Clear Them Up.
Misinterpretations of the “Cone of Uncertainty” in Florida during the 2004 Hurricane Season
Definition of the NHC Track Forecast Cone

Remember when a glass of wine a day was good for you? Here's why that changed. Popular Science, 10 September 2018

Googling the news
Economist, 1 September 2018

We sat in on an internal Google meeting where they talked about changing the search algorithm — here's what we learned

Reading , Writing and Risk Literacy

[1]

Today is the deadliest day of the year for car wrecks in the U.S.

Some math doodles

<math>P \left({A_1 \cup A_2}\right) = P\left({A_1}\right) + P\left({A_2}\right) -P \left({A_1 \cap A_2}\right)</math>

<math>P(E) = {n \choose k} p^k (1-p)^{ n-k}</math>

<math>\hat{p}(H|H)</math>

<math>\hat{p}(H|HH)</math>

Accidental insights

My collective understanding of Power Laws would fit beneath the shallow end of the long tail. Curiosity, however, easily fills the fat end. I long have been intrigued by the concept and the surprisingly common appearance of power laws in varied natural, social and organizational dynamics. But, am I just seeing a statistical novelty or is there meaning and utility in Power Law relationships? Here’s a case in point.

While carrying a pair of 10 lb. hand weights one, by chance, slipped from my grasp and fell onto a piece of ceramic tile I had left on the carpeted floor. The fractured tile was inconsequential, meant for the trash.

BrokenTile.jpg

As I stared, slightly annoyed, at the mess, a favorite maxim of the Greek philosopher, Epictetus, came to mind: “On the occasion of every accident that befalls you, turn to yourself and ask what power you have to put it to use.” Could this array of large and small polygons form a Power Law? With curiosity piqued, I collected all the fragments and measured the area of each piece.

Piece Sq. Inches % of Total
1 43.25 31.9%
2 35.25 26.0%
3 23.25 17.2%
4 14.10 10.4%
5 7.10 5.2%
6 4.70 3.5%
7 3.60 2.7%
8 3.03 2.2%
9 0.66 0.5%
10 0.61 0.5%
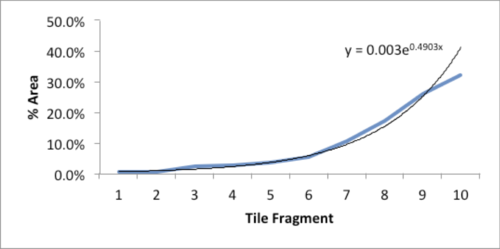
Montante plot1.png

The data and plot look like a Power Law distribution. The first plot is an exponential fit of percent total area. The second plot is same data on a log normal format. Clue: Ok, data fits a straight line. I found myself again in the shallow end of the knowledge curve. Does the data reflect a Power Law or something else, and if it does what does it reflect? What insights can I gain from this accident? Favorite maxims of Epictetus and Pasteur echoed in my head: “On the occasion of every accident that befalls you, remember to turn to yourself and inquire what power you have to turn it to use” and “Chance favors only the prepared mind.”

Montante plot2.png

My “prepared” mind searched for answers, leading me down varied learning paths. Tapping the power of networks, I dropped a note to Chance News editor Bill Peterson. His quick web search surfaced a story from Nature News on research by Hans Herrmann, et. al. Shattered eggs reveal secrets of explosions. As described there, researchers have found power-law relationships for the fragments produced by shattering a pane of glass or breaking a solid object, such as a stone. Seems there is a science underpinning how things break and explode; potentially useful in Forensic reconstructions. Bill also provided a link to a vignette from CRAN describing a maximum likelihood procedure for fitting a Power Law relationship. I am now learning my way through that.

Submitted by William Montante

Retrieved from "https://www.causeweb.org/wiki/chance/index.php?title=Sandbox&oldid=22455"